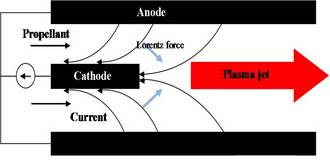
The present situation of space exploration calls for missions beyond the moon and for such missions, chemical propulsion is not a viable option, except for the case of launch vehicles where high thrust is required. Functionally, the inability of chemical propulsion systems to achieve higher exhaust velocities is due to limitation in the maximum tolerable temperature in the combustion chamber and to avoid excessive heat transfer to the walls. Both these limitations can be overcome by use of electric propulsion, which can be defined as the acceleration of gases for propulsion by electrical heating and/or by electric and magnetic volume forces. The magnetoplasmadynamic (MPD) thrusters have the unique capability, among all other developed electric propulsion systems, of processing megawatt power levels in a simple, small and robust device, producing thrust densities as high as 10⁵ N / m². These features render it an attractive option for high energy deep space missions requiring higher thrust levels than other electric thrusters. The specific impulse of a self-field MPD thruster is related to the parameter beta (the square of the discharge current divived by the mass flow rate), which is often used to characterize MPD thruster performance. High value of beta correspond to predominantly electromagnetic acceleration, and provide higher values of specific impulse. Low values of beta correspond to predominantly electrothermal acceleration, and lower values of specific impulse. MPD efficiency typically increases with increasing beta, but this also leads to strong numerical instabilities. This project deal with the modeling of the MPD thruster through a new approach, which consist to solve the compressible MHD equations in the density-based compressible flow solver platform available in the open source CFD package OpenFOAM. The main objectives of this project is to understand the complex nature of the coupled electromagnetic and gasdynamic acceleration processes and the effects of relevant flow-field parameters which are otherwise quite hard to analyse with experiments.
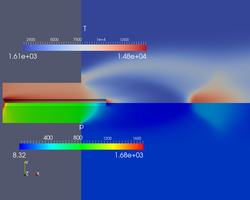
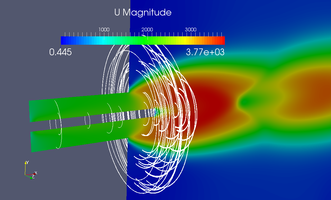